Graphs a function f(x) and the Taylor polynomial approximations to f(x) up to degree 4 for a given c value (the center point for the Taylor polynomials). .

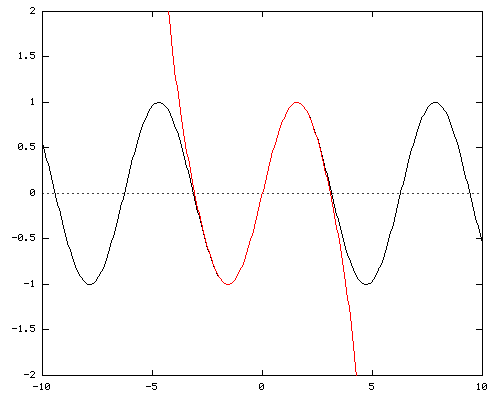The Taylor Series in $(x-a)$ is the unique power series in $(x-a)$ converging to $f(x)$ on an interval containing $a$. For this reason, .

Taylor Series Expansions in Graph and Table back to main page. — www.teacherlink .org . Taylor Series Expansions. x-axis .

This module illustrates Taylor series methods for numerically solving initial value problems for ordinary differential equations. A numerical method for an .

Get answers to your series expansion questions with interactive calculators. Solve Taylor, Laurent, or Puiseux series expansion problems.

A summary of Some Common Taylor Series in 's The Taylor Series. Learn exactly what happened in this chapter, scene, or section of The Taylor Series and what .

So, the Taylor Series approximation says that if I must approximate with a constant, the best approximation is the constant = . .

File Format: Microsoft Word - Quick View

Taylor and Maclaurin Series - Example 1 7 min - Apr 6, 2008 - Uploaded by patrickJMT

taylor(f) computes the Taylor series expansion of f up to the fifth order. The expansion point is 0 (Maclaurin series expansion). taylor(f, n) computes the .

For analytic functions the Taylor polynomials at a given point are finite order truncations of its Taylor's series, which completely determines the function .

Taylor and Maclaurin Series - Example 2 10 min - Apr 6, 2008 - Uploaded by patrickJMT

File Format: PDF/Adobe Acrobat - Quick View

A Taylor series approximates the chosen function. Move the locator to change the center of the approximation. Change the view to see the accuracy. .

On their intervals of convergences, Taylor series can be added, subtracted, and multiplied by constants and powers of x, and the resulting series will also .

Think of power series as "generalized" polynomials. Since (almost) all functions you encounter have a Taylor series, all functions can be thought of as .

This applet draws the graph of y = sin(x) along with its Taylor polynomial of the specified degree. Clicking the Draw button plots the polynomial of the .

Jun 10, 2007 . Derivation of Taylor Series Expansion. Objective: Given f(x), we want a power series expansion of this function with respect to a chosen .

Mar 24, 2011 . Taylor Series Remainder. A quantity that measures how accurately a Taylor polynomial estimates the sum of a Taylor series. .

File Format: PDF/Adobe Acrobat - Quick View

th-order Taylor series expansion of $ f(x)$ about the point $ x=0$ . . The main point to note here is that the Taylor series itself is simple to derive. .

May 10, 2011 . A Taylor series is a series expansion of a function about a point . st power are taken in the Taylor series (Whittaker and Watson 1990, pp .

File Format: PDF/Adobe Acrobat - Quick View

An online tool that will create a Taylor Series expansion of a function.

Taylor's series. We've seen in the last few sections that it can be useful to write functions as infinite series of powers of x, in particular this allows .

File Format: PDF/Adobe Acrobat - Quick View

In this second chapter on Taylor Series, we will be studying the case where the n.th derivative of an infinitely differentiable function, does not go to .

Find the Taylor series expansion of any function around a point using this online calculator.

www.ma.utexas.edu/cgi-pub/kawasaki/plain/infSeries/6.html - SimilarTaylor SeriesThis series is called the Taylor series of f at a . In the special case where a= 0, this is called the Maclaurin series . Of course, the statement "if f has .

In mathematics, a Taylor series is a representation of a function as an infinite sum of terms calculated from the values of its derivatives at a single .

File Format: Microsoft Powerpoint - Quick View

Taylor Series: Deriving the Series for exp(x) [YOUTUBE 5:30]. Taylor Series: Example: Finding sin(2.0) [YOUTUBE 10:32]. MULTIPLE CHOICE TESTS .

A Taylor series provides a way to generate such a series and is computed as: taylor series where f is the function for which we want a series representation .

A Category 2 or Category 3 power series in x defines a function f by setting for any x in the series' interval of convergence. The power series expansion .

There are many ways of coming up with the Taylor Series of a given function f(x) . Here are some recipes. (Note: in these recipes, all Taylor series are .

Taylor Series. In the previous section we started looking at writing down a power series representation of a function. The problem with the approach in that .

is a power series such that the radius of convergence is r > 0 then . Note that the first degree Taylor polynomial for f at x = c is the equation of the .

Nov 7, 2010 . The Taylor series of an infinitely often differentiable real (or complex) function f defined on an open interval (a-r, a+r) is the power .

Introduction to Taylor series and MacLaurin series. Also contains links to Taylor series expansions of exponential, logarithmic, trigonometric, .

Oct 18, 2008 . The Taylor series is mainly used for approximating functions when one can identify a small parameter. Expansion techniques are useful for .

File Format: PDF/Adobe Acrobat - Quick View

The methods discussed above have depended on the use of the Taylor series truncated after first order, either directly or in the evaluation of the mean .

Aug 23, 2008 . So how many terms should I use in getting a certain pre-determined accuracy in a Taylor series. One way is to use the formula for the .

File Format: PDF/Adobe Acrobat - Quick View

In general, $T$ may not extrapolate or approximate $f$ in the strictest sense: a Taylor series does not necessarily converge, and even if it does, .

File Format: PDF/Adobe Acrobat - Quick View

May 16, 2011 . Taylor Series gives us a good approximation for a function. This section shows how to find Taylor Series.

Diagrams of the Taylor series for sine (sinus) from the first power until the 17th power.

In fact, this demonstrates the use of Taylor series well: the function we have found . This means that the coeficients of the Taylor series are given by .

Sitemap